Decimal to Octal Converter
Looking for a quick and easy way to convert decimal to octal? Whether you’re a student tackling math problems or a tech enthusiast working on a coding project, converting decimal numbers to octal doesn’t have to be a headache. With Nam Generator’s Decimal to Octal Converter, you can get accurate results in just seconds—without the hassle!
Read More: Binary to Decimal Converter
What is Decimal to Octal Converter?
Before we dive into why our tool is the best choice, let’s clarify what Decimal to Octal Converter is. The decimal system (base 10) is what we use in everyday life—numbers are made up of digits from 0 to 9. The octal system (base 8), however, uses only digits from 0 to 7. Converting decimal to octal is essential in fields like computer science and digital electronics because some systems, like certain microcontrollers, rely on the octal number format.
Why Choose Nam Generator’s Converter?
Our Decimal to Octal Converter is designed to provide fast, accurate, and user-friendly results. Here’s why Nam Generator is your best choice:
- Instant Results: No more manual calculations! Simply enter your decimal number, and get the octal equivalent instantly.
- Easy to Use: No tech expertise needed—our converter is designed for everyone, from students to professionals.
- Free & Accessible: Available anytime, anywhere! Whether you’re on your desktop or mobile, our free converter is at your service.
- Error-Free Calculations: Enjoy peace of mind knowing that your conversions are 100% accurate.
How to Use Nam Generator’s Decimal to Octal Converter
Using our tool is as easy as 1-2-3:
- Enter Your Decimal Number: Type your decimal number into the converter box.
- Click Convert: Hit the convert button and watch the magic happen!
- Get Your Octal Number: Instantly see your result and use it for your projects, studies, or personal needs.
Why Decimal to Octal Converter?
Converting decimal to octal has various practical applications, particularly in computer programming, networking, and digital hardware design. Octal numbers are more compact than binary numbers, making them easier to manage in some contexts. Whether you’re learning about number systems in school or working with octal values in coding, our converter will save you time and effort.
How to Decimal to Octal Converter
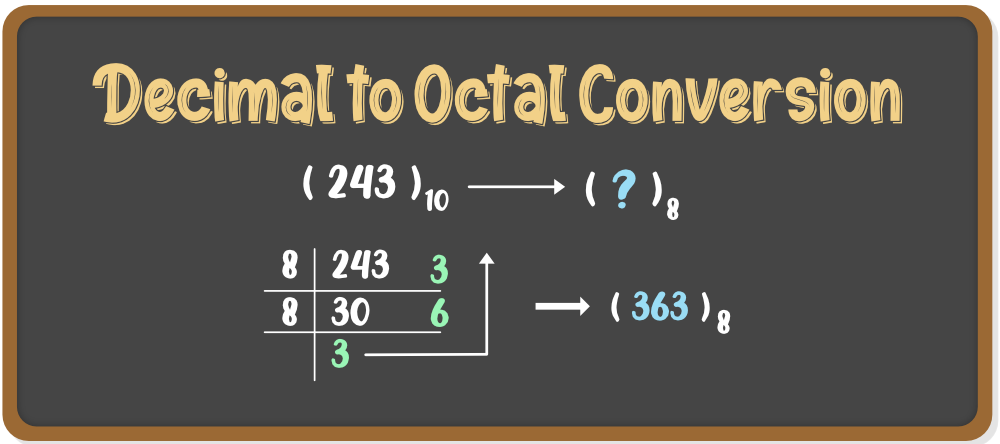
Decimal to Octal Converter can be achieved by applying the repeated division and remainder algorithm. Simply put, the decimal number is repeatedly divided by the radix 8. In between these divisions, the remainders give the octal equivalent in reverse order.
Here is how to Decimal to Octal Converter step by step:
- Step 1: If the given decimal number is less than 8, the octal equivalent is the same. If the given number is greater than 7, divide the number by 8.
- Step 2: Write down the remainder.
- Step 3: Divide the part before the decimal point of your quotient by 8 again.
- Step 4: Write down the remainder.
- Step 5: Continue this process of dividing by 8 and noting the remainders until the last decimal digit you are left with is less than 8.
- Step 6: When the last decimal digit is less than 8, the quotient will be less than 0 and the remainder will be the digit itself.
- Step 7: The last remainder you get will be the most significant digit of your octal value while the first remainder from Step 3 is the least significant digit. Therefore, when you write the remainders in reverse order – starting at the bottom with the most significant digit and going to the top- you will reach the octal value of the given decimal number.
Decimal to Octal Converter Chart Table
| Decimal | Octal |
|---|---|
| 1 | 1 |
| 2 | 2 |
| 3 | 3 |
| 4 | 4 |
| 5 | 5 |
| 6 | 6 |
| 7 | 7 |
| 8 | 10 |
| 9 | 11 |
| 10 | 12 |
| 11 | 13 |
| 12 | 14 |
| 13 | 15 |
| 14 | 16 |
| 15 | 17 |
| 16 | 20 |
| 17 | 21 |
| 18 | 22 |
| 19 | 23 |
| 20 | 24 |
| 21 | 25 |
| 22 | 26 |
| 23 | 27 |
| 24 | 30 |
| 25 | 31 |
| 26 | 32 |
| 27 | 33 |
| 28 | 34 |
| 29 | 35 |
| 30 | 36 |
| 31 | 37 |
| 32 | 40 |
| 33 | 41 |
| 34 | 42 |
| 35 | 43 |
| 36 | 44 |
| 37 | 45 |
| 38 | 46 |
| 39 | 47 |
| 40 | 50 |
| 41 | 51 |
| 42 | 52 |
| 43 | 53 |
| 44 | 54 |
| 45 | 55 |
| 46 | 56 |
| 47 | 57 |
| 48 | 60 |
| 49 | 61 |
| 50 | 62 |
| 51 | 63 |
| 52 | 64 |
| 53 | 65 |
| 54 | 66 |
| 55 | 67 |
| 56 | 70 |
| 57 | 71 |
| 58 | 72 |
| 59 | 73 |
| 60 | 74 |
| 61 | 75 |
| 62 | 76 |
| 63 | 77 |
| 64 | 100 |
| Decimal | Octal |
|---|---|
| 65 | 101 |
| 66 | 102 |
| 67 | 103 |
| 68 | 104 |
| 69 | 105 |
| 70 | 106 |
| 71 | 107 |
| 72 | 110 |
| 73 | 111 |
| 74 | 112 |
| 75 | 113 |
| 76 | 114 |
| 77 | 115 |
| 78 | 116 |
| 79 | 117 |
| 80 | 120 |
| 81 | 121 |
| 82 | 122 |
| 83 | 123 |
| 84 | 124 |
| 85 | 125 |
| 86 | 126 |
| 87 | 127 |
| 88 | 130 |
| 89 | 131 |
| 90 | 132 |
| 91 | 133 |
| 92 | 134 |
| 93 | 135 |
| 94 | 136 |
| 95 | 137 |
| 96 | 140 |
| 97 | 141 |
| 98 | 142 |
| 99 | 143 |
| 100 | 144 |
| 101 | 145 |
| 102 | 146 |
| 103 | 147 |
| 104 | 150 |
| 105 | 151 |
| 106 | 152 |
| 107 | 153 |
| 108 | 154 |
| 109 | 155 |
| 110 | 156 |
| 111 | 157 |
| 112 | 160 |
| 113 | 161 |
| 114 | 162 |
| 115 | 163 |
| 116 | 164 |
| 117 | 165 |
| 118 | 166 |
| 119 | 167 |
| 120 | 170 |
| 121 | 171 |
| 122 | 172 |
| 123 | 173 |
| 124 | 174 |
| 125 | 175 |
| 126 | 176 |
| 127 | 177 |
| 128 | 200 |
| Decimal | Octal |
|---|---|
| 129 | 201 |
| 130 | 202 |
| 131 | 203 |
| 132 | 204 |
| 133 | 205 |
| 134 | 206 |
| 135 | 207 |
| 136 | 210 |
| 137 | 211 |
| 138 | 212 |
| 139 | 213 |
| 140 | 214 |
| 141 | 215 |
| 142 | 216 |
| 143 | 217 |
| 144 | 220 |
| 145 | 221 |
| 146 | 222 |
| 147 | 223 |
| 148 | 224 |
| 149 | 225 |
| 150 | 226 |
| 151 | 227 |
| 152 | 230 |
| 153 | 231 |
| 154 | 232 |
| 155 | 233 |
| 156 | 234 |
| 157 | 235 |
| 158 | 236 |
| 159 | 237 |
| 160 | 240 |
| 161 | 241 |
| 162 | 242 |
| 163 | 243 |
| 164 | 244 |
| 165 | 245 |
| 166 | 246 |
| 167 | 247 |
| 168 | 250 |
| 169 | 251 |
| 170 | 252 |
| 171 | 253 |
| 172 | 254 |
| 173 | 255 |
| 174 | 256 |
| 175 | 257 |
| 176 | 260 |
| 177 | 261 |
| 178 | 262 |
| 179 | 263 |
| 180 | 264 |
| 181 | 265 |
| 182 | 266 |
| 183 | 267 |
| 184 | 270 |
| 185 | 271 |
| 186 | 272 |
| 187 | 273 |
| 188 | 274 |
| 189 | 275 |
| 190 | 276 |
| 191 | 277 |
| 192 | 300 |
| Decimal | Octal |
|---|---|
| 193 | 301 |
| 194 | 302 |
| 195 | 303 |
| 196 | 304 |
| 197 | 305 |
| 198 | 306 |
| 199 | 307 |
| 200 | 310 |
| 201 | 311 |
| 202 | 312 |
| 203 | 313 |
| 204 | 314 |
| 205 | 315 |
| 206 | 316 |
| 207 | 317 |
| 208 | 320 |
| 209 | 321 |
| 210 | 322 |
| 211 | 323 |
| 212 | 324 |
| 213 | 325 |
| 214 | 326 |
| 215 | 327 |
| 216 | 330 |
| 217 | 331 |
| 218 | 332 |
| 219 | 333 |
| 220 | 334 |
| 221 | 335 |
| 222 | 336 |
| 223 | 337 |
| 224 | 340 |
| 225 | 341 |
| 226 | 342 |
| 227 | 343 |
| 228 | 344 |
| 229 | 345 |
| 230 | 346 |
| 231 | 347 |
| 232 | 350 |
| 233 | 351 |
| 234 | 352 |
| 235 | 353 |
| 236 | 354 |
| 237 | 355 |
| 238 | 356 |
| 239 | 357 |
| 240 | 360 |
| 241 | 361 |
| 242 | 362 |
| 243 | 363 |
| 244 | 364 |
| 245 | 365 |
| 246 | 366 |
| 247 | 367 |
| 248 | 370 |
| 249 | 371 |
| 250 | 372 |
| 251 | 373 |
| 252 | 374 |
| 253 | 375 |
| 254 | 376 |
| 255 | 377 |
Decimal to Octal
The Decimal to Octal Converter involves transforming numbers from the decimal system, which is base-10, into the octal system, which is base-8. While the decimal system uses ten digits (0 to 9), the octal system only uses eight digits (0 to 7). Decimal numbers are often Decimal to Octal Converter to octal for specific computing and programming needs, as octal is closely related to binary, making it easier to process by machines. This is a crucial function in many mathematical calculations and digital systems.
Hexadecimal to Octal Converter
In addition to decimal, numbers in the hexadecimal system (base-16) can also be converted to octal. Hexadecimal uses sixteen symbols (0-9 and A-F), where A stands for 10, B for 11, and so on, up to F for 15. To perform a hexadecimal-to-octal conversion, numbers must first be converted from hexadecimal to binary, then from binary to octal. Online Hexadecimal to Octal converters are available to facilitate this process by quickly converting values without the need for manual calculations.
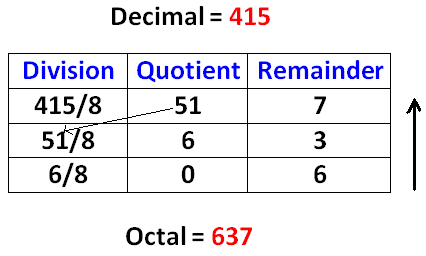
Decimal to Octal Converter Formula
The formula for Decimal to Octal Converter involves repeatedly dividing the decimal number by 8 and recording the remainder. The steps are:
- Divide the decimal number by 8.
- Write down the remainder.
- Divide the quotient from the previous division by 8 again.
- Continue dividing until the quotient becomes zero.
- The octal equivalent is the sequence of remainders read from bottom to top.
Example: Convert the decimal number 78 to octal:
- 78 ÷ 8 = 9 (remainder: 6)
- 9 ÷ 8 = 1 (remainder: 1)
- 1 ÷ 8 = 0 (remainder: 1)
Thus, 78 in decimal is 116 in octal.
Decimal to Octal Table
To simplify conversion, decimal to octal tables provide a quick reference for commonly converted numbers. Below is a sample of a decimal to octal table:
| Decimal | Octal |
|---|---|
| 0 | 0 |
| 1 | 1 |
| 2 | 2 |
| 3 | 3 |
| 4 | 4 |
| 5 | 5 |
| 6 | 6 |
| 7 | 7 |
| 8 | 10 |
| 9 | 11 |
| 10 | 12 |
| … | … |
| 100 | 144 |
This table makes it easy to find octal equivalents for smaller decimal numbers. For larger numbers, a calculator is usually more efficient.
Decimal to Octal with Decimal Point
Converting decimal numbers with fractional parts (numbers with decimal points) to octal follows a slightly different process. Here’s how:
- Convert the integer part of the decimal number to octal using the standard method.
- Multiply the decimal (fractional) part by 8.
- The integer part of the result is the next digit in the octal representation.
- Repeat the multiplication until the fractional part becomes zero or reaches a satisfactory precision.
Example: Convert 45.625 to octal:
- Integer part: 45 in decimal converts to 55 in octal.
- Fractional part: 0.625 × 8 = 5.000, so the fractional part in octal is .5.
Thus, 45.625 in decimal is 55.5 in octal.
Decimal to Octal Questions
- What is 34 in octal?
- To convert 34 to octal, divide by 8: 34 ÷ 8 = 4 remainder 2. So, 34 in decimal is 42 in octal.
- How do you convert a fractional decimal to octal?
- Convert the integer part using the division method and multiply the fractional part by 8 repeatedly to get the octal fraction.
- What is the use of the octal system?
- The octal system is primarily used in computing and digital systems because of its close relation to binary.
Decimal to Octal Converter Examples PDF
For in-depth study, you can download a Decimal to Octal Converter Examples PDF that includes step-by-step explanations and exercises for converting both whole numbers and fractions. This resource is especially useful for students and professionals looking for practice problems or reference material for quick conversions. You can find downloadable PDFs on educational websites or create your own with various solved examples for future reference.
Octal Calculator
An Octal Calculator simplifies the process of converting decimal numbers to octal. These calculators are available online and can instantly convert decimal numbers to octal with or without decimal points. They are handy tools for quick calculations, making them indispensable for programmers, engineers, and students. The calculators are designed to handle both simple conversions and more complex calculations involving large numbers.
Conclusion:
The Decimal to Octal Converter is a valuable tool that transforms numbers from the decimal system into the octal system, a process important in digital computing and electronics. Whether you’re working with whole numbers or fractional decimal points, using a manual method or an online calculator, understanding the fundamentals of Decimal to Octal Converter can enhance your grasp of numerical systems used in technology.
Read More: Decimal to Octal Converter
FAQs:
What is a Decimal to Octal Converter?
A tool that converts decimal (base-10) numbers into octal (base-8) numbers.
How do you convert decimal to octal manually?
Divide the decimal number by 8 and record the remainders, then read them from bottom to top.
Why is the octal system used in computers?
The octal system simplifies binary coding, as each octal digit represents exactly three binary digits.
What is 25 in decimal converted to octal?
25 in decimal is 31 in octal.
Can I convert decimal fractions to octal?
Yes, by converting the integer and fractional parts separately using division and multiplication by 8.
What are common applications of the octal system?
Used in computing, especially in low-level programming and digital electronics.
Is there a direct method for converting hexadecimal to octal?
No, you first convert hexadecimal to binary, then binary to octal.
Can negative decimal numbers be converted to octal?
Yes, convert the absolute value to octal and add the negative sign.
What is 100 in decimal in the octal system?
100 in decimal is 144 in octal.
What tools are available for Decimal to Octal Converter?
You can use online converters, calculators, or manual methods.
What’s the difference between binary and octal systems?
Binary uses two digits (0, 1), while octal uses eight digits (0-7).
How does a calculator help in Decimal to Octal Converter?
A calculator automates the division and remainder process, providing quick results.
Is there a Decimal to Octal Converter app?
Yes, several apps and websites offer instant conversion tools for decimal to octal.
Can I convert octal numbers back to decimal?
Yes, you can reverse the process by multiplying each digit by powers of 8.
What is the octal equivalent of 78?
The octal equivalent of 78 in decimal is 116.
How do I convert 0.625 decimal to octal?
Multiply the fractional part (0.625) by 8 to get 5, so 0.625 in octal is .5.
What is the octal number system?
A base-8 number system that uses digits 0 to 7.
Can I use Decimal to Octal Converter in programming?
Yes, it’s often used in programming, particularly in dealing with permissions in UNIX-based systems.
What’s the octal equivalent of 45.5 in decimal?
45.5 in decimal is 55.4 in octal.
Is converting large decimal numbers to octal complicated?
For very large numbers, it’s easier to use an octal calculator or converter to avoid errors.